Irrational Number

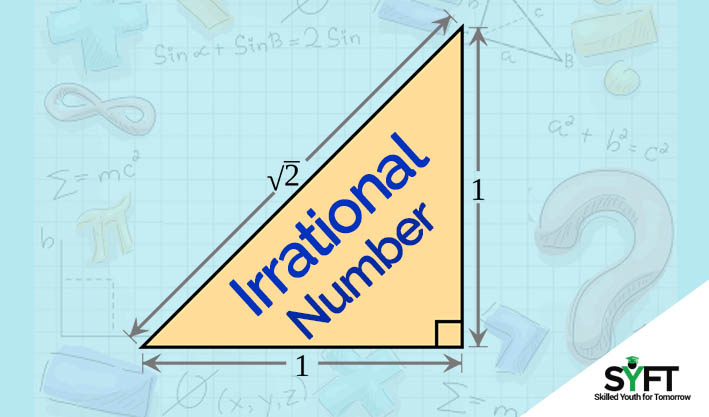
Prove that \(\sqrt{2}\) is irrational.

Solution: If not, let us assume   \(\sqrt{2}\) is rational.

Then \(\sqrt{2}\)  =p/q (q ≠0 , (p,q) ≠ 2)

=> 2 = p²/q²

 ^=>  2 q²=  p²

 Now 2 q² is divisible by 2, hence p² is divisible by 2

Then p is divisible by 2. Now let p = 2r

2q² = 4 r²

q² = 2 r²

q² is divisible by 2.

q is divisible by 2

It contradicts our assumption that p and q are not divisible by 2.

Prove that is \(\sqrt{3}\)irrational.

Solution: If not, let us assume \(\sqrt{3}\) is rational.

Then \(\sqrt{3}\) =p/q (q ≠0 , (p,q) ≠ 3)

=> 3 =p²/q²

 ^=> 3q²=  p²

Now 3q² is divisible by 3, hence p² is divisible by 3

Then p is divisible by 3. Now let p=3r

3q² = 9 r²

q² = 3 r²

q² is divisible by 3.

q is divisible by 3

It contradicts our assumption that p and q are not divisible by 3.

Prove that  \(\sqrt{5}\) is irrational.

Solution: If not, let us assume \(\sqrt{5}\) is rational.

Then  \(\sqrt{5}\)  =p/q (q ≠0 , (p,q) ≠ 5)

=>5 =p²/q²

5q²=  p²

Now 5 q² is divisible by 5, hence p² is divisible by 5

Then p is divisible by 5. Now let p=5r

5q² = 25 r²

q² = 5 r²

q² is divisible by 5.

q is divisible by 5

It contradicts our assumption that p and q are not divisible by 5.