Approximate Value
When we round off decimal to some digit, all digits after this digit will be replaced by zero, and if they are after the dot, then they will be deleted. If the first next digit after this digit is more than or equal 5, then the last remaining digit will be not changed.
Example 1.
We should round off a=2471.05624 accurate to within tens.
Digit of unit next to position of tenths equals 1, i.e. is less than 5. So, rounding off to tens, we have a=2470.
The symbol of “=” is called the sign of approximate equality.
Example 2.
We should round off a=2471.05624 accurate to within units.
Digit of tenths equals 0, so, rounding off to units, we have a=2471.
Example 3.
We should round off a=2471.05624 accurate to within tenths.
Digit of tenths equals 5, so, rounding off to units, we have 2471.1.
Example 4.
We should round off a=2471.05624 accurate to within hundredths.
Digit of hundredths equals 6, so, rounding off to units, we have a=2471.06 .
Example 5.
We should round off a=2471.05624 accurate to within thousandths.
Digit of ten thousandths equals 2, so, rounding off to thousandths, we have a=2471.056.
All of the values, that was found in above examples are called approximate values of the number a.
Suppose a -approximate values of the number a. Then the absolute value of difference of number a and a , i.e. |a−a| is called absolute error of approximate values of the number, and ratio of absolute error to the absolute value of approximate value is called relative error of approximate value. The relative error is denominated in percents.
Example 6.
When we weigh the detail, the mass of which is 54.12705 gram, on the scales with the scale division 0.1 gram, we obtain approximate value of mass 54.1 gram. We should find absolute and relative errors of this approximate value.
The absolute error equals 54.12705−54.1=0.02705 ; the relative error equals (0.02705/54.1) ×100=0.05 %.
If absolute error of approximate value a, which we have found for the number a that are interested us, does′t exceed some number h, then we write a=a ± h; and we say, that a – is approximate value a accurate within h.
Example 7.
We should find the approximate value of the number a=2471.05624 accurate within 0.01.
Rounding off the number accurate within hundredths, we will obtain a=2471.06. Absolute error of this approximate value equals |2471.05624−2471.06|−|0.00376|<0.01. So, 2471.06 – approximate value of the number accurate within 0.01.
Approximation theory is one of the parts of mathematics. Approximation is used when it is difficult to find the exact value of any number.
It is also used to round off the errors leading to approximation.
Symbol of Approximation
Generally we use the wavy equal “≈” sign to represent the approximate values which mean “almost equal to”.
Example
π ≈ 3.14
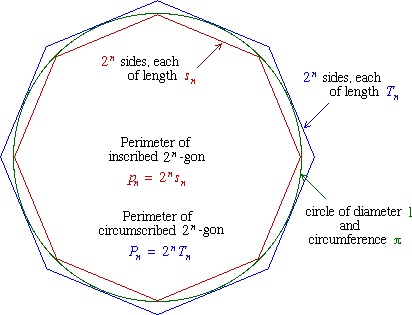
Approximation of pi
Pi is the ratio of the circumference of a circle to its diameter. This is irrational so it has an infinite amount of decimal places.
So the approximation of pi or = 3.14
Approximation of pi by Various Mathematicians
There are so many mathematicians who had given their contribution in finding the approximation of pi.
The method of Archimedes involves approximating pi by the perimeters of polygons inscribed and circumscribed about a given circle